What is time dilation?

Time dilation is one of the most important results of “Special theory of relativity”. In fact, it is the weirdest result of “Special theory of relativity” which we never experience in our daily life. But how weird and impossible it seems to be, it is verified in all the experiments. All the experiments give the same result. Even it is experienced all the time in nuclear reactions. So, let us understand “time dilation” in the simplest way, with the spacetime diagrams. Here again we will assume the speed of light to be exactly 1. If you are wondering how the speed of light is exactly 1 when its value is (), go through the article “can the speed of light be exactly 1?”.  So time and all the spatial coordinates are measured in meters. Now consider the following diagram.

Suppose and are two observers.  moves with uniform velocity v(which is assumed to be near the speed of light) with respect to the observer . The world line of  is the t axis(which means that  is rest in his frame as he does not move in his frame though time is passing) and world line of  is axis i.e.  moves along the axis in the spacetime diagram. Note that  moves along the axis in the both frame but the fact is that  is at rest in  frame but is in motion in 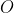 frame.

 Here you can see that the coordinate systems of the two observers are different which means that the coordinates of events measured by 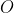 and  are different. They will measure different time coordinate for the same event. Different observers calculate different readings for the same event. For example, in two dimensional coordinate system, different coordinate systems measure different positions(i.e. different values of x and y coordinates) of the same point( see the figure below).

The same thing happens in 4 dimensional spacetime. Different observers measure different coordinate values(including the time coordinate) for the same event. Now consider the following diagram –

To understand time dilation, you should have a basic knowledge of what interval in spacetime is. Consider the two dimensional coordinate system(fig 2). Different systems measure different coordinate values but notice one thing that the distance between the two points does not change. It is fixed and does not depend on coordinate system. Similarly for the three dimensional systems, different coordinate systems measure different values of the coordinates but the distance between the two points does not change. The same is applicable in four dimensional spacetime. The distance between the two events does not depend on the coordinate system. This four dimensional distance is called the “interval”. Interval is defined by the following equation –

  is the interval. Notice that there is a negative sign for the time coordinate. This should not be confused with the Euclidean three dimensional space.

Suppose,  and 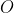 both have clocks which are set to zero at point X and then started. Now, consider the point A at which ’s clock measure 1m(Note that time is measured in meter). Will the observer 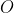 also measure the same time? No! Say, 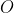 measures t time at event A. As we know, the interval between two events is observer independent. Then the interval between X and A is –





Now,   is the time measured by  and all the other coordinates are zero(as  is at rest in his frame). So, the second equation becomes,

Now,   and  are zero and  =   so,





So, the calculations and the diagram suggest that the value of  the time measured by the observer  is greater than the time measured by the observer . In general,



You can see that  is always greater than  and it totally depends on the velocity of   with respect to . Note that observer  is observing 's 1m time for a greater time. It means that in  frame, when  reaches 1m time,  has already covered 1m time. This is called "Time Dilation".